Quantum Field Theory (QFT) is the current theory of how atomic and subatomic particles behave. It is the most up-to-date mathematical formulation of quantum mechanics (as of this writing in 2017).
QFT can be used to predict the results of quantum physics experiments with great accuracy, possibly the greatest accuracy of any scientific theory. Take, for example, the calculation of the value of the fine structure constant. 1 The fine structure constant is a constant of nature important in the quantum world. QFT can be used to calculate the value of this constant to an accuracy of 11 places beyond the decimal point: .00729735256. This accuracy has been verified by comparing it with experimental results.
This article describes the key elements of Quantum Field Theory.
The most fundamental entities of matter or energy are quantum fields.
For example, there is a quantum electromagnetic field, a quantum electron field, a quantum up-quark field, etc. Each field fills the entire universe and has a value at every point in the universe. By “value” is meant the strength of the field at that point.

As fields are the most fundamental entities in the universe that we know of, we can’t say anything further about their ingredients, what substance they might be made of.
In the accompanying diagram, the red netting represents the underlying quantum field. A localized 3-dimensional wave travels through it. The macroscopic level of reality, where we detect the associated particle, is shown in green. The particle is shown as an orange or blue circle. A sequence of “snapshots” (Diagrams A, B, C, D) show a 3-dimensional wave traveling in the (red netting) field: (A) the wave crests and then, (B) forms a trough, (C) crests again, and (D) forms a second trough. The green film represents the macroscopic level of reality in which we detect particles. A particle is shown traveling towards the center of the green film from the lower corner. The particle manifests the underlying wave and is the entity that we detect. We cannot detect the field itself. In Quantum Field Theory, the particle is the manifestation of the deeper reality of a localized wave traveling through a field.
Our knowledge of quantum fields is limited to mathematical equations.
We have not seen nor heard nor felt quantum fields. Our knowledge of them is limited to mathematical equations which describe the fields and predict the results of experiments in the quantum world.
Quantum fields are, in a sense, physical.
Quantum fields are physical in the sense that they create real, lawful effects in spacetime. For this reason, their behavior is verifiable in physics experiments. However, quantum fields do not exist in spacetime in the same way that tables and chairs exist. If quantum fields existed in spacetime, we would be required to agree with paradoxical statements that make quantum mechanics seem baffling:
- Electrons exist in more than one place at the same time.
- Electrons shoot from an electron gun as a particle, travel as a wave, and land on a detector as a particle.
- Electrons don’t have definite properties until they have an interaction with another part of the physical universe.
- Particles travel backwards in time as in the delayed choice two-slit experiment.
It may be more understandable to think of quantum fields as existing in a sublevel of reality, which Dr. Ruth Kastner calls, “Quantumland.” (Then again, it may not seem more understandable!)
Quantumland is governed by the laws of quantum physics. It is represented by the red netting in the four figures higher up in this article; whereas, spacetime is represented by the green film. Dr. Kastner writes, “Yet despite the amenability of quantum systems to public verifiability, …quantum systems are not contained within spacetime. It is this ‘in-between-ness’ that leads to the proposed interpretation of quantum systems as a new form of possibility which is physically real but which transcends the spacetime realm.” 2 By “in-between-ness,” Kastner means in-between the idea level and a real physical thing in spacetime.
Quantumland and the analogy of a video game.
Not everyone will find the video game analogy helpful. If it loses you, just move on to the next point.
We can think of Quantumland on analogy with an on-line computer program for a video game. The computer program is analogous to Quantumland (red netting in the diagrams above), and the visuals on the computer screen are analogous to events in spacetime (green film). Let’s say that the video game is the story of Sleeping Beauty. The programmer, whom we’ll name…uh…”Programmer,” codes the game to include three alternative story lines:
- Sleeping Beauty is never kissed and sleeps on into eternity,
- The prince kisses her lovingly and she wakes up, and
- Sleeping Beauty is kissed but not with love, only with lust, and dies!
These are analogous to possible outcomes of a two-slit experiment: the particle can travel through Slit A, Slit B, or both.
Later, Programmer’s girlfriend, named…uh…”Playeress,” plays the game. Playeress clicks away trying to get the prince to kiss Beauty, but watches in dismay as the prince walks right past Beauty without kissing her. This alternative takes on a reality that the two other alternatives lack–it’s acted out on the screen. The two other alternatives, present in the computer code, never make it to the screen.
This is analogous to the way that Quantumland’s many possibilities take on only one physical reality. In the double-slit experiment, of the three possibilities, only one, let’s say, a particle traveling through Slit A, shows up in the physical universe.
Playeress can’t reach back in time and undo the prince’s failure to kiss Beauty. Maybe Playeress can get herself awarded a re-do, but the fact of the matter is that in her first round, the kiss was missed.
But let’s say that Programmer wants to give Playeress a second chance without her having to earn a re-do. Programmer re-writes the code on-line so that Playeress’s first-round clicks are deleted. Now, the program no longer includes the code that led up to and includes the missed kiss. In the view of Playeress, her clicks were miraculously deleted. It’s as if something later in time has caused effects on something earlier in time.
This is similar to backwards-in-time quantum effects as in the delayed choice double-slit experiment. This analogy can also cast light on the notion that photons don’t experience time at all–they seem to live in stopped time. Just as computer code acts like a sublevel to the story that plays on the screen, Quantumland acts like a sublevel to physical reality.
Waves travel through quantum fields.
Quantum fields vibrate in the same sense that air vibrates with sound waves when a bell is rung. What we call a “particle” is the manifestation of a localized vibration in a quantum field. Where the electron field is vibrating, we can detect an electron. Where the electromagnetic field is vibrating, we can detect a photon. (Photons are the particles associated with the electromagnetic field.) For more detail, watch this excellent five-minute video https://youtu.be/FBeALt3rxEA?t=3m28s.
Fields explain why all particles of the same type are identical.
Electrons are a manifestation of a vibration of the electron field. This is why all electrons are identical. All electrons have exactly the same amount of mass, charge, and spin–properties that an electron is “born” with. When placed in the same conditions, all electrons have exactly the same potential for traveling and interacting. Electrons are interchangeable with each other just as photons are interchangeable with each other. This is because all particles of the same type are manifestations of vibrations in the same field. The field has certain properties–shake the field at the right frequency, and in that locality you get a particle having the properties of the field.
Fields explain why high energies create particles.
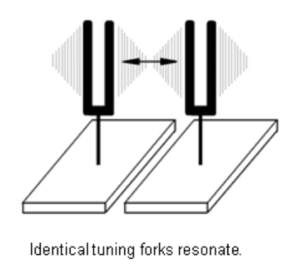
In other words, the field is pre-existing. When physicists “create a particle,” they are shaking the right field by hitting it with the frequency that it resonates with. This is like striking one Middle C tuning fork, which causes the nearby air to vibrate, which, in turn, sets another Middle C tuning fork to vibrating. As shown in the accompanying diagram, just the right frequency of vibrating air can set the second tuning fork vibrating. Similarly, the right frequency can set a quantum field to vibrating.
Vibrating a particular field, let’s say the Higgs field, causes the physical manifestation of a Higgs particle. In the case of the Higgs field, it vibrates when a very high frequency is reached. In the quantum world, frequency is proportional to energy, so very high energies are needed to create a Higgs particle.
However, the physicists needn’t know how to “make a particle,”—how to manipulate its properties to make sure it has the right mass, spin, etc. The Higgs field “knows” all that—when it’s shaken at the right frequency, a wave is set up, and a Higgs particle manifests and can be detected.
Every particle has its own quantum field.
Both fundamental particles, like electrons, and composite particles, like protons, have their own unique field. In addition to the fields for electromagnetism and electrons, there is a neutrino field, a neutron field, a Higgs field, etc. In 2012, when the discovery of the Higgs particle was announced, quantum physicists often described it among themselves as finding the “Higgs field,” rather than the “Higgs particle.” Because much of the public is not familiar with fields, the news media called it a “boson,” a type of particle.
Quantum fields interact.
When physicists observe particles being emitted or absorbed, what’s happening at the field level? One field is setting off a vibration in another field. Another way to say this is that energy from one field is being transferred to another field. For example, when the electromagnetic field vibrates, it can set the electron field vibrating. This would be detected as a photon being absorbed by an electron (the photon being the particle which manifests the electromagnetic field).
Energy transfers between fields are in discrete packets.
When the electromagnetic (EM) field sets the electron field vibrating, it transfers a packet of energy, a quantum, to the electron field. When viewing this as a particle interaction, we would say that the EM field gives the electron field a photon (a quantum of EM energy). The EM field cannot give ½ a quantum nor .0794 of a quantum nor any other fractional amount, but only 1 full quantum, one photon. This is a distinguishing property of quantum fields—energy transfers must be in discrete packets or lumps (quanta) and cannot be in continuous amounts.
This contrasts with the macroscopic world of tables and chairs. In the macroscopic world, energy transfers from one object to another in arbitrary amounts, not lumps of specific sizes. It’s as if in the quantum world, energy transfers are completed as if energy were bricks. Whereas in the macroscopic world, energy transfers are completed as if energy were water—just measure out any amount.
QFT–Field interactions or particle interactions?
In the case of the electromagnetic field, the energy transfer is a transfer of photons. Many physicists prefer to think of a photon as a particle rather than as a wave traveling through a field. So, they describe everything in terms of particles.

In Feynman Diagrams, the position of the particle is represented on the horizontal axis and time is represented on the vertical axis. Straight lines represent the trajectories of particles of matter, like electrons, and squiggly lines represent the trajectories of force-carrying particles, like photons.
The accompanying Feynman Diagram shows an exchange of a virtual photon (squiggly line) between two electrons (straight lines). A virtual particle is defined as an extremely short-lived particle. An electron (green trajectory) emits a virtual photon, recoils, and then heads off on a new (green) trajectory. If the energy of the photon is just right, it will be absorbed by another electron. In this case, a second electron (on a blue trajectory) absorbs the photon, which throws it off course onto a second (blue) trajectory. 4
Taken together, this is the meaning of the entire interaction: Two electrons, particles with like electrical charges, repel each other. Thus, they head towards each other, exchange a virtual photon, and then head away from each other. In this interaction, virtual photons carry or “mediate” the electrical charge. This is a typical interaction described by QFT.
Other physicists prefer to conceptualize QFT in terms of waves or vibrations in fields. Frank Wilczek, who won the Nobel Prize for his discoveries in nuclear physics, often takes this approach. One of the advantages of the field view is that it easily allows visualization of why all particles of the same type (electrons, for example) are identical—the same field is being vibrated. This view also makes it more understandable as to how physicists can “create particles” which have all the right attributes just by creating high energies in accelerators. Physicists are not really creating anything, they are shaking a pre-existing field.
However, since physicists find it difficult to explain fields to lay people, they often speak only of particles in presentations to lay audiences.
Quantum fields are in continuous motion.
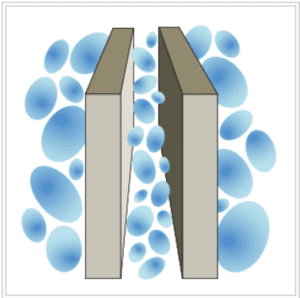
When physicists take the particle view, they describe these fluctuations as the appearance and disappearance of virtual particles. Virtual particles are like real particles except that they are extremely short-lived. They pop into existence and blink out in tiny fractions of a second. For example, an electron and an anti-electron can pop into existence, then both blink out of existence. The result would be minute fluctuations in the energy level of the electron field.
While empty space is empty of real particles, that is, those which remain in existence indefinitely, it is full of virtual particles that briefly appear and disappear. These appearances and disappearances are accompanied by tiny fluctuations of the energy levels of the affected quantum fields.
A very brief history of QFT.


In 1973, physicists developed the mathematical equations describing the interactions of quarks and gluons. These are interactions which describe the behavior of protons and neutrons in the nucleus of the atom. This theory is named “Quantum Chromodynamics” (QCD) to echo the name “Quantum Electrodynamics” (QED). Quantum Chromodynamics is also part of Quantum Field Theory.
Today, Quantum Field Theory includes QED, QCD, and mathematical equations which describe many other field interactions, including those of the Higgs field. Quantum Field Theory is the most recent mathematical formulation of quantum mechanics. However, earlier versions of quantum mechanics, Schrodinger’s Wave Equation and Heisenberg’s Matrix Mechanics, continue in use. Each version has its own area of application, but QFT is the most complete and accurate approach.
Footnotes
